Part 41: The 0.463% Reality
Part 41: The 0.463% Reality
Back to the Rec Room, until we hit on that 1/216 chance...
I mentioned last time that the Rec Room was my favorite puzzle room.
That's true, but I'm less of a fan of the rest of the fragment. Not so much because of "The 99.537% Reality" ending here, though.
When I first played this game, the Rec Room was actually the first fragment I played once things opened up.

As a puzzle room it was great. As an introduction into the structure of the rest of the game, not so much.

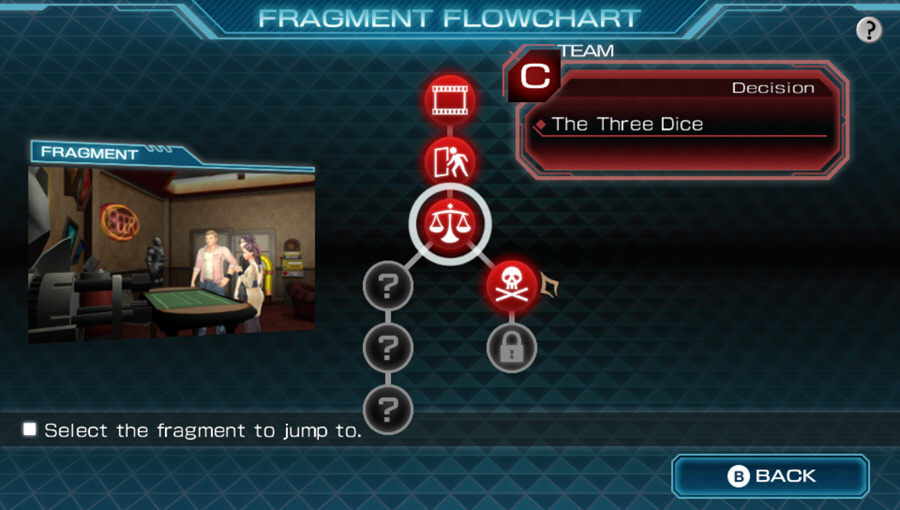
First, we have the decision that isn't really a decision.

Then, we are faced with incredibly poor odds, that are almost certain to demotivate a new player.

After failing the first time, what would possibly motivate someone to try again? Particularly when there's a lock shown on the fragment afterwards.

Maybe you'd try one more time, just to see what would happen.

But it's still just as likely to produce the same result.

And at that point, you're even less likely to try yet a third time.

Maybe I'm missing something, you'd think.

Maybe I'll just come back to this later, you'd say to yourself. There are plenty of other fragments, after all.

Unfortunately, no matter what, you'll still end up facing these dice again.

You see, most of the locked fragments remaining will not become available until we get all "1"s here.

And not only is there no indication of that fact, but there's also no indication that you're facing anything other than a grueling slog of replaying this fragment, potentially hundreds of times, in order to achieve the desired result.

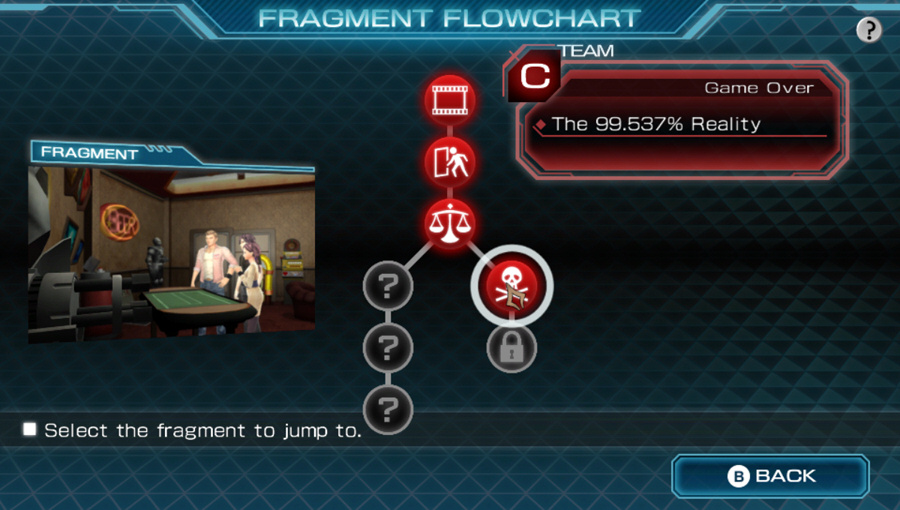
Fortunately, after watching C-Team fail twice, all the way through the "Game Over" screen, on the third time...

...the game takes pity on us, forcing us down the other branch.

So now that we've seen "The 99.537% Reality" a couple of times, let's see what awaits us in "The 0.463% Reality".
Music: Clarification 2nd Mix



The guns retract, unfired, and the door slides open.





 We just got a miracle!
We just got a miracle!

Junpei's reaction is how the player would feel by getting this result naturally. Carlos and Akane react the way the player would, knowing it was rigged.

 We're...alive, right?
We're...alive, right? Of course we're alive!
Of course we're alive!
 What, do I look like a ghost to you?! Or an undead zombie?! If I was, then my heart wouldn't be pounding a mile a minute right now!
What, do I look like a ghost to you?! Or an undead zombie?! If I was, then my heart wouldn't be pounding a mile a minute right now!
Junpei saunters off to the exit.

 Come on, let's blow this popsicle stand!
Come on, let's blow this popsicle stand!
 Come on! What are you doing?! Hurry up!
Come on! What are you doing?! Hurry up!


 I'm really glad...
I'm really glad... That we're alive? Or...
That we're alive? Or... Both...
Both...Music: Placidity 2nd Mix



 Come on, you guys should join me.
Come on, you guys should join me.






 You probably shouldn't drink too much, Junpei...
You probably shouldn't drink too much, Junpei... Why not? We're just going to conk out again in 40 minutes anyway, remember?
Why not? We're just going to conk out again in 40 minutes anyway, remember?
No, the amount of time taken to do the puzzle room doesn't factor into this time. It does happen to be close in this case, though.

 We just experienced the miracle of hitting 1/216 odds! 0.46%! Guys, come on!
We just experienced the miracle of hitting 1/216 odds! 0.46%! Guys, come on!
 Let's use the remaining time we have to savor this awesome moment!
Let's use the remaining time we have to savor this awesome moment!
 It definitely would be, if it happened on the first try...
It definitely would be, if it happened on the first try... Guys, what are you saying? We only threw the dice once, right?
Guys, what are you saying? We only threw the dice once, right?
 Are you sure about that...? Maybe we just don't remember, and in reality we've rolled the dice over and over again.
Are you sure about that...? Maybe we just don't remember, and in reality we've rolled the dice over and over again.Akane's either breaking the fourth wall or accessing the morphogenetic field. Come to think of it, I'm not sure how much difference there really is between the two.

Music: Sinisterness Phase 2


I don't know, still pretty long odds there.

 So what? Does that really matter?
So what? Does that really matter?
 I'll say it again; we've only gone and thrown the dice once. Once.
I'll say it again; we've only gone and thrown the dice once. Once.

 What are you trying to say, Akane?
What are you trying to say, Akane?
 Carlos, let's say you were going to roll ten dice all at the same time. So, do you think you'd end up with all of them displaying a "1"?
Carlos, let's say you were going to roll ten dice all at the same time. So, do you think you'd end up with all of them displaying a "1"?

 The probability is 60,466,176 to 1, but that's essentially impossible. If you tried 60,466,176 times, however, what would happen?
The probability is 60,466,176 to 1, but that's essentially impossible. If you tried 60,466,176 times, however, what would happen?Akane is able to figure out 6^10 pretty quickly, too. Statistics don't mean you're guaranteed to get 10 "1"s in that many tries (it has about a 63% chance of happening at least once over that whole set, if I did my math right), but it does make it much more likely.
 The likelihood of all of them being 1 is...
The likelihood of all of them being 1 is...So, it's not really what they're getting at here, but the formula comes from probability theory for what are called Bernoulli trials (like rolling dice some number of times): (1 - (60,466,175 / 60,466,176) ^ 60,466,176) * 100% = 63.2%, plus some change. That seems like a pretty specific number, given how many trials are involved. In fact, the odds of hitting a 1-in-"n" chance over the course of "n" trials happens to be (1 - 1/e)*100% = 63.2...%, when "n" gets sufficiently large like it does here. If you don't remember what "e" is, you had a better time in school than I did.

63.2% isn't a sure thing for having to do that many trials, but I agree it's much better than impossible.
 I'll give you another example. Junpei. If 2 to the 4th power -- 16 -- people compete in a rock, paper, scissors tournament,
I'll give you another example. Junpei. If 2 to the 4th power -- 16 -- people compete in a rock, paper, scissors tournament, 
Bet you didn't expect this update to turn into a math lesson. This question isn't quite worded right - if there are 16 people and one person is eliminated with each match, then of course you need 15 total matches to determine the winner. But what she's really asking, is how many matches would a single individual have to win in a row in order to win the tournament?

Junpei objects to being given math problems while drunk. I promise there's a point to all this.

 Just answer the question.
Just answer the question.
Junpei sighs, then talks out loud while he figures it out.

 Uh, really? Okay, first the 16 narrow down to 8, then 8 to 4, and 4 to 2... After the final round, one person's left standing, so... A total of 4?
Uh, really? Okay, first the 16 narrow down to 8, then 8 to 4, and 4 to 2... After the final round, one person's left standing, so... A total of 4?
 So, what would happen if 2 to the 100 did the same thing?
So, what would happen if 2 to the 100 did the same thing?2^100 is some crazy 31-digit number. Odds of any given individual winning, if they all had a 50% chance at each round, would be 7.89*10^-29 percent. Very very low. I think that's the same odds of flipping a coin 100 times and it coming up heads every single time. Exponential growth is a bitch.


 I'm not, obviously.
I'm not, obviously.
 You're out of the competition after you lose just one match. You'd have to win a hundred rounds straight to win.
You're out of the competition after you lose just one match. You'd have to win a hundred rounds straight to win.Seems impossible, right? And yet...

There's always a winner. Impossible odds for each individual at the beginning, and yet someone always wins.

 A victor who wins a hundred matches without losing one will exist.
A victor who wins a hundred matches without losing one will exist.Kind of like how in the multiverse theory of this series, no matter how unlikely the outcome, there is some version of C-Team that would achieve it. Just because someone had to be the winner.

 Do you know what I'm trying to say?
Do you know what I'm trying to say?



 "The one who lives on does so due to circumstance."
"The one who lives on does so due to circumstance."Basically, there's no point marveling over the fact that they got lucky and lived, because some version of the team had to get lucky and live, and since they're the ones around to observe the results, it must have been them. This concept is called...


 Yeah, it's connected to the many-worlds and multiverse theories...
Yeah, it's connected to the many-worlds and multiverse theories...

 That principle explains why.
That principle explains why.
 They say, for example, that if Jupiter was just a bit smaller, the Earth likely wouldn't have even formed. That's not the only instance either. There are physical constants throughout the universe, but if even one had changed the tiniest bit, humanity wouldn't have been created at all.
They say, for example, that if Jupiter was just a bit smaller, the Earth likely wouldn't have even formed. That's not the only instance either. There are physical constants throughout the universe, but if even one had changed the tiniest bit, humanity wouldn't have been created at all.

 We exist, therefore we know that we exist.
We exist, therefore we know that we exist.One group uses the fact that the evolution of life is so unlikely to have happened to mean that God must have created/engineered it. The counter-argument is that no matter how unlikely it might have been, in an infinite number of universes, the one we're observing must exist and meet the conditions necessary for human existence because that's the only way we could be observing it.

 Perhaps somewhere in reality there are countless universes devoid of humanity, where the idea of humans didn't even begin. But it isn't possible to know if those other universes exist or not.
Perhaps somewhere in reality there are countless universes devoid of humanity, where the idea of humans didn't even begin. But it isn't possible to know if those other universes exist or not.
 That's because there are no human beings to observe within them. The only universe we can measure is our own... Therefore this is the universe we exist in.
That's because there are no human beings to observe within them. The only universe we can measure is our own... Therefore this is the universe we exist in.

 The left and right sides of the equation say the same thing.
The left and right sides of the equation say the same thing.



 Maybe somewhere in this world, there are an infinite number of histories where we were executed by the gatling guns. But we don't know if those histories really exist. Basically, because we're dead in those histories. The dead can't observe the world. Only those who are still alive can do that... Therefore, we exist here. Now.
Maybe somewhere in this world, there are an infinite number of histories where we were executed by the gatling guns. But we don't know if those histories really exist. Basically, because we're dead in those histories. The dead can't observe the world. Only those who are still alive can do that... Therefore, we exist here. Now.
Small nod from Akane. What a good student Carlos is!

I think Junpei is already hungover.

The rest of this scene goes a little off the rails but is still all

 The universe could be not meant for humanity. Maybe there's one not limited by gravity, where they travel faster than light... You can't say for sure such a universe doesn't exist. If it does, then we could expect that aliens would be interacting together within it.
The universe could be not meant for humanity. Maybe there's one not limited by gravity, where they travel faster than light... You can't say for sure such a universe doesn't exist. If it does, then we could expect that aliens would be interacting together within it.

 Maybe that was the universe God had intended in the first place...
Maybe that was the universe God had intended in the first place...
 And this one is a failure...
And this one is a failure...

Junpei stands up and slams his beer down.
 What's the matter?
What's the matter?You're a buzzkill, Akane, that's what's the matter.

 You guys talking like this... I don't feel like drinking anymore.
You guys talking like this... I don't feel like drinking anymore.

 I mean, don't you agree?
I mean, don't you agree?

 Then maybe it's really true...
Then maybe it's really true...
 We might be in the history God abandoned.
We might be in the history God abandoned.Music: Bereavement

Was wondering when computer lady would chime in.





[Comptuer Lady:] That is all.
Q-Team's X-Passes are the same ones we saw in the Infirmary and the Trash Disposal Room. MOON for Diana is different...a VLR tie-in maybe? And FATE for Sigma is probably because he's trying to change his own future/fate.

 No...
No... That means only Phi is still alive...
That means only Phi is still alive...
 Sorry.
Sorry.

 Seems like we're the ones with the best luck. God hasn't gone and abandoned us!
Seems like we're the ones with the best luck. God hasn't gone and abandoned us!
I see we're back to asshole-Junpei.

 Didn't you hear that announcement?
Didn't you hear that announcement?
 The only one left to die is Phi from D-Team, and then--
The only one left to die is Phi from D-Team, and then--
A slap, and the screen turns white.

 Junpei...
Junpei...
 Don't you ever say that. Even if it's a joke...
Don't you ever say that. Even if it's a joke...
Akane walks away.


Junpei reaches into his pocket.

Yeah, probably not the best time for that.


And a slow fade to black.
By now you probably recognize that it's only when we fade to static that we've actually reached the end of a fragment. Not having done that, means...
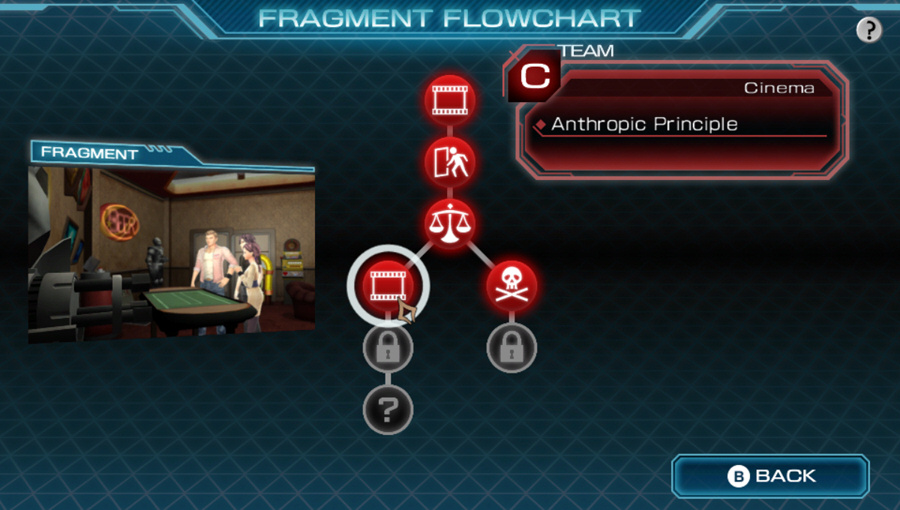
Yup, there's a lock on both branches.
However! I skipped past something important on the fragment select screen...

C-Team has a new fragment! Second row on the left. It gets unlocked by a complicated set of conditions that amount to having seen six particular scenes, including the one we just did. Can't really tell much from the image, but the only room we don't have a fragment for in Ward C yet is the Power Room, so I'll guess it's that one. And I'll add it to the poll!
Speaking of which, we are up to eight new fragments again (four of which we just unlocked in the last few updates), and still have five fragments left to be replayed. Where to next? Select the story fragment.